- Given a binary tree, count number of nodes using non recursive algorithm.
- We will calculate size of binary tree using breadth first search (bfs) or level order traversal algorithm.
- During binary tree traversal, we will keep on adding the number of elements at each level.
- At end of traversal, we will get number of nodes in a binary tree.
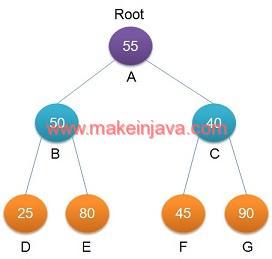
Algorithm: count number of nodes in a binary tree using java(Fig 1)
- Create local variable nNodes (signify the node count)
- nNodes initialized to 0
- Visit the Level 0
- visit all nodes in Level 0 and found only node A
- nNodes increment by 1 [nNodes = 1]
- Go to Level 1 and visit all nodes in Level 1
- Found node B, nNodes increment by 1 [nNodes = 2]
- Found node C, nNodes increment by 1 [nNodes = 3]
- Go to Level 2 and visit all nodes in Level 2
- Found node D, nNodes increment by 1 [nNodes = 4]
- Found node E, nNodes increment by 1 [nNodes = 5]
- Found node F, nNodes increment by 1 [nNodes = 6]
- Found node G, nNodes increment by 1 [nNodes = 7]
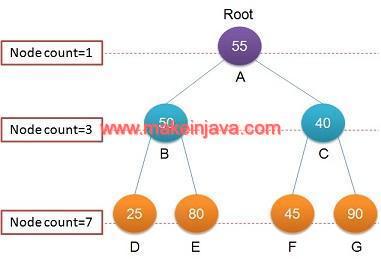
- Visited all nodes in binary tree [Refer Fig 2]
The time complexity of algorithm is O(n).
Program – number of nodes or size of a binary tree using java (BFS)
1.) CountNodes Class:
- Find number of nodes or size of a binary tree.
- Traverse binary tree using level order traversal.
package org.learn.Question;
import java.util.LinkedList;
import java.util.Queue;
public class CountNodes {
public static int countNodes(Node root) {
if (root == null) {
System.out.println("Tree is empty");
return -1;
}
int nNodes = 0;
Queue<Node> queue = new LinkedList<Node>();
queue.offer(root);
while (!queue.isEmpty()) {
Node node = queue.poll();
if (node.left != null) {
queue.offer(node.left);
}
if (node.right != null) {
queue.offer(node.right);
}
nNodes++;
}
System.out.println("Number of nodes in a binary tree : " + nNodes);
return nNodes;
}
}
2.) Node Class:
- Node class is representing the nodes of a binary tree.
package org.learn.Question;
public class Node {
public int data;
public Node left;
public Node right;
public Node(int num) {
this.data = num;
this.left = null;
this.right = null;
}
public Node() {
this.left = null;
this.right = null;
}
public static Node createNode(int number) {
return new Node(number);
}
}
3.) App Class:
- We are creating a binary tree in main method
- We are calling method of CountNodes class, to find number of nodes in a binary tree.
package org.learn.Client;
import org.learn.Question.CountNodes;
import org.learn.Question.Node;
public class App {
public static void main(String[] args) {
// root level 0
Node A = Node.createNode(55);
// Level 1
Node B = Node.createNode(50);
Node C = Node.createNode(40);
// Level 2
Node D = Node.createNode(25);
Node E = Node.createNode(80);
Node F = Node.createNode(45);
Node G = Node.createNode(90);
// connect Level 0 and 1
A.left = B;
A.right = C;
// connect level 1 and level 2
B.left = D;
B.right = E;
C.left = F;
C.right = G;
CountNodes.countNodes(A);
}
}
Output – Size of binary tree using level order traversal using java
Number of nodes in a binary tree : 7
Download code – size of binary tree non recursive algorithm in java